The Poisson Distribution

1  Introduction

If a random event occurs with known average over a fixed interval (this may be time, distance, area or volume), the Poisson Distribution can be used to calculate the probability of a number of these random events occuring over an interval. If the number of these events is , the discrete probability distribution is given by

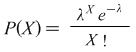
where is the exponential constant and is the mean value of the distribution.

1.1  Example

i) A bus service runs from a bus stop on average every 12 minutes. What is the probability of 3 buses arriving over a period of 24 minutes?

Solution:

The average number of buses over a 24 minute period is buses. The probability of 3 buses arriving during a 24 minute interval is therefore given by

.

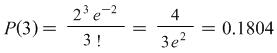ii) Another bus service runs on average every 6 minutes. What is the probability of 2 or more buses arriving at the stop in a 9 minute period?

Solution:

The probability of 2 or more buses arriving is .

The average number of buses over a 9 minute period is buses.

The probability of 0 buses arriving at the stop over a 9 minute period is given by

.

The probability of 1 bus arriving at the stop over a 9 minute period is given by

.

The probability of 2 or more buses arriving is therefore .

2  Mean, variance and standard deviation of the Poisson Distribution

The mean of a Poisson distribution is given by .

The variance of a Poisson distribution is given by .

The standard deviation of a Poisson distribution is given by .

3  Moment generating function of the Poisson Distribution

The moment generating function of the Poisson dsitribution is given by

.